Fundamentals of Fluid Flow in Porous Media

 

Chapter 2

Multi-phase Saturated Rock Properties:

Saturation History

We will be able to understand capillary phenomena in porous media much better if we see how the capillary pressure changes in some elementary geometry. Assume there are one wetting and one non-wetting fluid in the system that is axial-symmetric so the two radii of curvature are equal. Consider first Figure 2‑48 where a non-wetting fluid enters a circular pore from a bulk reservoir. The capillary pressure will increase with volume until it reaches a limiting value of 2σ/R (R is the principle radius and is equal to r/cosθ , eq.(2‑83)). In other word the capillary tube could be displaced completely by the non-wetting fluid if:

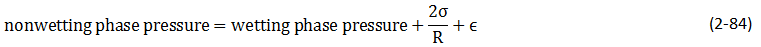
Where, ε is a very small value.

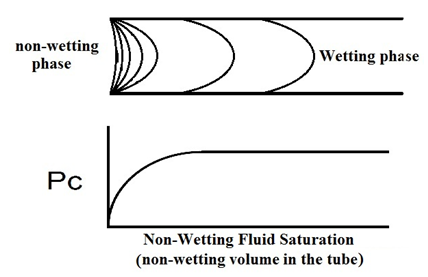
Figure 2-48: Non-Wetting Fluid Entering the Capillary Tube

The next example is a capillary tube with varying diameter. Capillary pressure increases by decreasing the tube diameter, eq.(2‑78). So, the capillary pressure increases by decreasing the wetting phase saturation and the required pressure to displace the wetting phase increases step by step (Figure 2‑49).

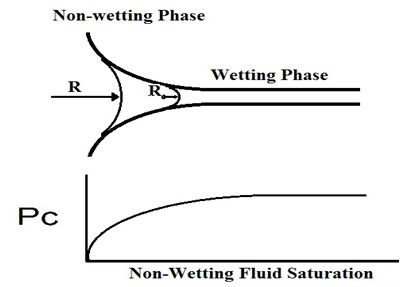
Figure 2-49: Non-Wetting Fluid Entering the Non-Uniform Capillary Tube

Another example is a non-wetting fluid exiting a circular pore and entering a large reservoir filled with the wetting fluid as in Fig. 5.2. This is similar to blowing bubbles from a glass straw. Suppose the interface is flat at the exit of the pore after the previous bubble detached. If the non-wetting phase is pumped with a controlled volumetric rate, the pressure will first increase as the interface increases in a curvature from zero (flat) to that of a hemisphere. The pressure will then decrease as the interface expands into a surface of a growing sphere. If the non-wetting fluid was a reservoir with an increasing pressure rather than at a constant volumetric rate, the bubble (or drop) will first grow slowly corresponding to the rate of pressure increase until it reaches the maximum pressure upon reaching the hemispherical shape and then it will suddenly grow in size (Figure 2‑50). By continuing the non-wetting injection and reducing the interface curvature, capillary increases to reach to its maximum at the entrance of the second capillary tube.

Figure 2-50: Non-Wetting Fluid Entering Entering & Exiting a Bubble